Next: 7 行列式の計算 Up: 4 行列式 Previous: 5 行列式の行に関する性質 Contents
6 行列式の列に関する性質
定理 4.44 (転置行列の行列式)
| (656) |
問 4.45 (転置行列の行列式)
これを示せ.
定理 4.46 (行列式の列に関する性質)
行列式は次の性質もつ.
- (1)
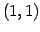 成分を除いて
成分を除いて 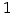 行目が全て 0 の場合は
行列式のサイズが一つ下がる.
行目が全て 0 の場合は
行列式のサイズが一つ下がる.
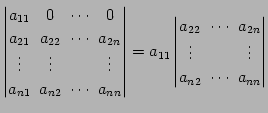
(657)
- (2)
- 第
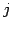 列行目の要素全てが共通因子
列行目の要素全てが共通因子 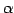 をもつとき,
をもつとき,
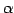 は行列式の外へ.
は行列式の外へ.
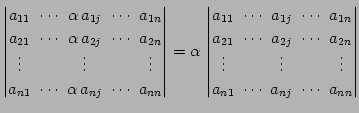
(658)
- (3)
- 第
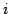 列が二つのベクトルの和で表されるとき,
行列式の和に分解される.
列が二つのベクトルの和で表されるとき,
行列式の和に分解される.
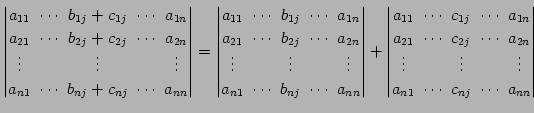
(659)
- (4)
- 第
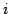 列と第
列と第 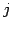 列を入れ替えると
行列式の符合が反転する.
列を入れ替えると
行列式の符合が反転する.
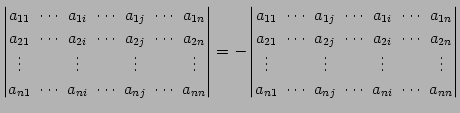
(660)
- (5)
- 同じ列があるときは行列式は 0 となる.
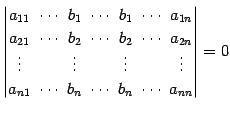
(661)
- (6)
- 第
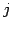 列を
列を 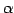 倍して
第
倍して
第 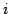 列に加えても行列式は等しい.
列に加えても行列式は等しい.
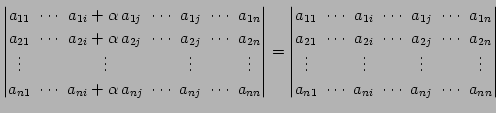
(662)
問 4.47
これを示せ.
Next: 7 行列式の計算 Up: 4 行列式 Previous: 5 行列式の行に関する性質 Contents
Kondo Koichi

Created at 2004/11/26