2.21 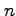 変数関数と 1 変数関数の合成関数の微分
変数関数と 1 変数関数の合成関数の微分
定理 2.94 (多変数関数の合成関数の微分)
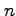 変数関数
変数関数
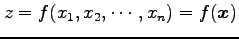 と
1 変数関数
と
1 変数関数
の合成関数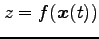 の微分は
の微分は
で与えられる. ここで
とおくと,
と書ける. また, 代入も含めて書くと
となる.
の合成関数
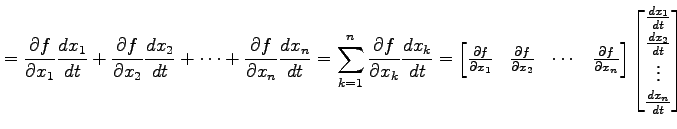 |
で与えられる. ここで
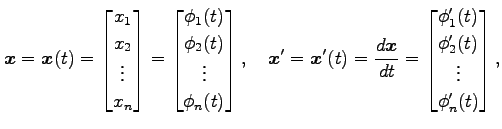 |
||
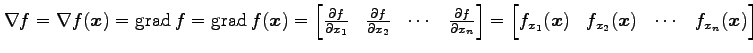 |
とおくと,
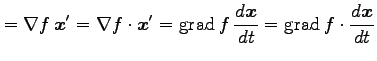 |
と書ける. また, 代入も含めて書くと
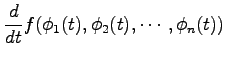 |
||
となる.
注意 2.95 (多変数関数の合成関数の微分)
曲面
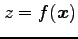 の点
の点 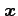 における
法線ベクトルは
における
法線ベクトルは
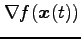 である(接平面の節を参照).
である(接平面の節を参照).
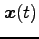 の軌跡は
の軌跡は
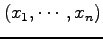 空間内の曲線である.
この曲線の接ベクトルは
空間内の曲線である.
この曲線の接ベクトルは
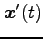 である.
である.
平成21年1月14日