3.3 導関数の計算
定理 3.8 (微分演算に関する性質)
関数 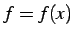 ,
, 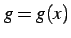 が微分可能なとき,
次の関係が成り立つ:
が微分可能なとき,
次の関係が成り立つ:
- (1)
- (和の微分)
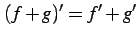 .
.
- (2)
- (微分の線形性)
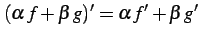 (
(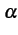 ,
, 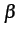 :定数).
:定数).
- (3)
- (積の微分)
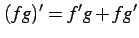 .
.
- (4)
- (商の微分)
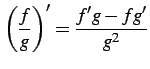 (
(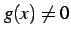 ).
).
- (5)
- (合成関数の微分)
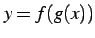 のとき
のとき 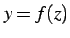 ,
, 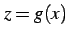 とおけば
とおけば
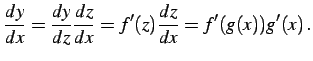
(204)
この演算規則をチェインルール(chain rule)と呼ぶ. - (6)
- (逆関数の微分)
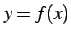 ,
,
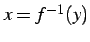 のとき
のとき
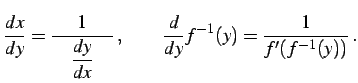
(205)
例 3.9 (導関数の計算例)
次の関数の導関数を求めよ.
| (206) | ||
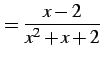 |
(207) | |
| (208) | ||
| (209) |
問 3.10
微分演算に関する性質を示せ.
を得る.
を得る.
を得る.
を得る. ここで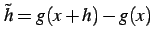 とおくと,
とおくと,
となる.ここで
が成り立つ.よって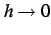 のとき
のとき
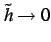 である.
以上より
である.
以上より
を得る.
となる.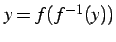 の両辺は
の両辺は 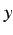 に関する関数である.
両辺を
に関する関数である.
両辺を 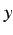 で微分すると
で微分すると
を得る.よって
となる.
(証明)(1)
![]() とおく.
定義に従い計算すると
とおく.
定義に従い計算すると
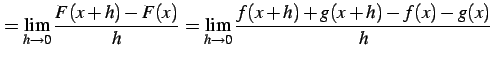 |
||
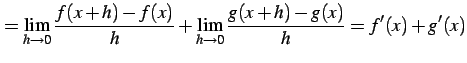 |
(210) |
を得る.
(2)
![]() とおく.
定義に従い計算すると
とおく.
定義に従い計算すると
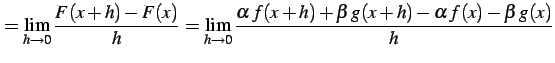 |
||
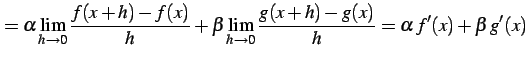 |
(211) |
を得る.
(3)
![]() とおく.定義に従い計算すると
とおく.定義に従い計算すると
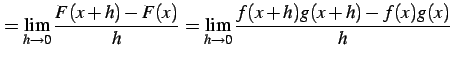 |
||
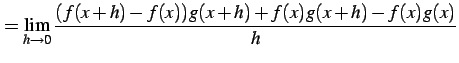 |
||
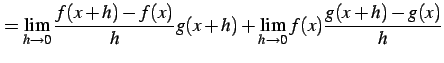 |
||
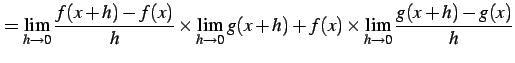 |
(212) | |
| (213) |
を得る.
(4)
![]() とおく.定義に従い計算すると,
とおく.定義に従い計算すると,
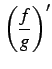 |
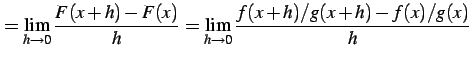 |
(214) |
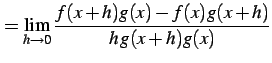 |
(215) | |
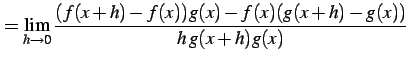 |
(216) | |
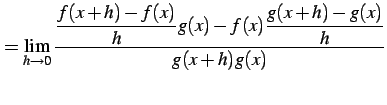 |
(217) | |
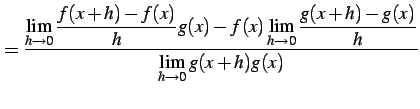 |
(218) | |
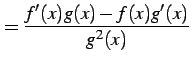 |
(219) |
を得る.
(5)
![]() とおく.定義に従い計算すると
とおく.定義に従い計算すると
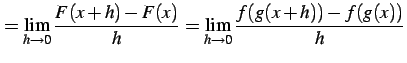 |
||
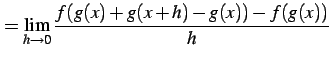 |
||
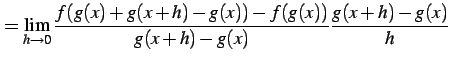 |
(220) |
を得る. ここで
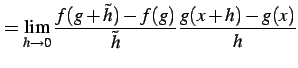 |
||
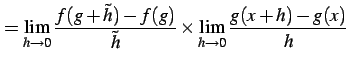 |
(221) |
となる.ここで
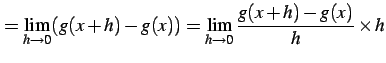 |
||
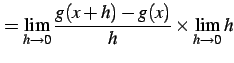 |
||
| (222) |
が成り立つ.よって
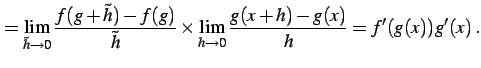 |
(223) |
を得る.
(6) ![]() ,
,
![]() より
より
| (224) |
となる.
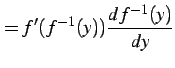 |
(225) | |
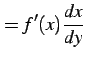 |
(226) | |
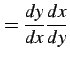 |
(227) |
を得る.よって
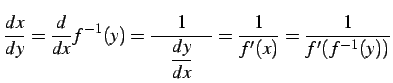 |
(228) |
となる.
Kondo Koichi

平成17年8月31日