Next: 16 連続関数 Up: 2 関数 Previous: 14 関数の極限 Contents
15 連続と不連続
定義 2.60 (関数の連続性)
次の条件を満たすとき,関数 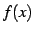 は点
は点 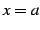 において
連続(continuous)であるという.
において
連続(continuous)であるという.
- (i)
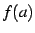 が定義されている.
が定義されている.
- (ii)
-
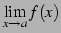 が存在する.
が存在する.
すなわち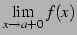 と
と
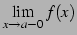 が存在し,それらの値が等しい.
が存在し,それらの値が等しい.
- (iii)
-
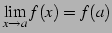 が成立する.
が成立する.
すなわち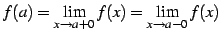 が
成立する.
が
成立する.
例 2.61 (連続な点の具体例)
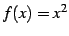 は
は 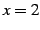 において連続である.
なぜなら
において連続である.
なぜなら
が成り立つからである.
| (302) |
が成り立つからである.
例 2.62 (不連続な点の具体例)
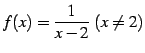 は
は
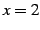 において不連続である.
なぜなら
において不連続である.
なぜなら 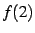 は定義されていない.
さらには
は定義されていない.
さらには
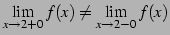 となるからである.
となるからである.
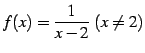 は
は
例 2.63 (不連続点の除去の具体例)
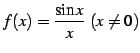 は
は 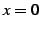 において
不連続である.なぜなら
において
不連続である.なぜなら 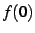 が定義されていないからである.
しかし
が定義されていないからである.
しかし 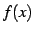 を
を
と定義すると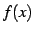 は
は 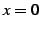 において連続となる.
なぜなら
において連続となる.
なぜなら
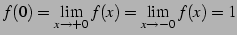 が
成立するからである.
再定義することにより不連続な点
が
成立するからである.
再定義することにより不連続な点 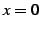 は取り除かれた.
は取り除かれた.
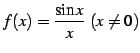 は
は ![$\displaystyle f(x)=\left\{ \begin{array}{cc} \displaystyle{\frac{\sin x}{x}} & (x\neq 0)\\ [1em] 1 & (x=0) \end{array} \right.$](img1041.png) |
(303) |
と定義すると
例 2.64 (不連続点を除去できない具体例)
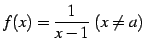 は点
は点 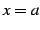 において不連続である.
点
において不連続である.
点 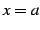 における値を
における値を 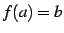 と定義することにする.
うまく
と定義することにする.
うまく 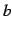 を定めることにより不連続点は取り除くことができるであろうか.
を定めることにより不連続点は取り除くことができるであろうか.
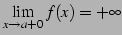 ,
,
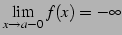 であるので,
点
であるので,
点 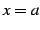 の左右で極限がことなる.よってどのように
の左右で極限がことなる.よってどのように 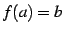 を定めても
不連続な点を取り除くことはできない.
を定めても
不連続な点を取り除くことはできない.
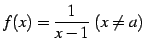 は点
は点
例 2.65 (不連続点の除去の具体例)
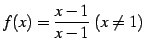 を考える.
を考える.
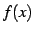 は点
は点 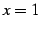 において不連続である.
しかし
において不連続である.
しかし 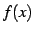 は分子分母が等しいので,
は分子分母が等しいので, 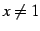 となる点において
となる点において
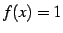 である.よって
である.よって
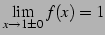 となる.
ゆえに点
となる.
ゆえに点 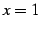 の値を
の値を
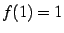 と定義すれば不連続点は取り除かれる.
結局,点
と定義すれば不連続点は取り除かれる.
結局,点 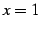 はみかけ上の不連続点であり本質的な不連続点ではない.
はみかけ上の不連続点であり本質的な不連続点ではない.
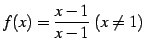 を考える.
を考える.
問 2.66
参考書(p.36)問題 2-5.
問 2.67 (不連続点の除去の例)
次の関数を 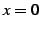 で連続となるように
で連続となるように 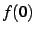 の値を定義せよ.
の値を定義せよ.
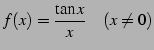 |
(304) | |
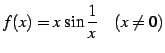 |
(305) | |
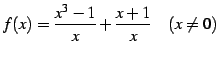 |
(306) |
Next: 16 連続関数 Up: 2 関数 Previous: 14 関数の極限 Contents
Kondo Koichi
Created at 2003/08/29