1 行列式の導出

連立一次方程式

$$\left\{ \begin{array}{ccccc} a_{11}\,x_{1} & + & a_{12}\,x_{2} & = & b_{1} \\ a_{21}\,x_{1} & + & a_{22}\,x_{2} & = & b_{2} \end{array} \right.$$ (528)

を考える． このときこの方程式が一意な解ともつ条件を求める． 方程式を書き直すと

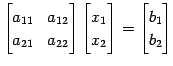$$\begin{bmatrix}a_{11} & a_{12} \\ a_{21} & a_{22} \end{bmatrix} \... ...atrix}x_{1} \\ x_{2} \end{bmatrix}= \begin{bmatrix}b_{1} \\ b_{2} \end{bmatrix}$$ (529)

となる． 拡大係数行列は

$$[A\,\vert\,\vec{b}] = \left[ \begin{array}{cc\vert c} a_{11} & a_{12} & b_{1} \\ a_{21} & a_{22} & b_{2} \end{array} \right]$$ (530)

である． 簡約化を行う：

$$\left[ \begin{array}{cc\vert c} a_{11} & a_{12} & b_{1} \\ a_{21} & a_{22} & b_{2} \end{array} \right]$$ (531)

$$-a_{21}/a_{11}$$ (532)

$$\longrightarrow \left[ \begin{array}{cc\vert c} a_{11} & a_{12} &... ...}}} & \displaystyle{\frac{a_{11}b_{2}-b_{1}a_{21}}{a_{11}}} \end{array} \right]$$ (533)

$$a_{11}$$ (534)

$$\longrightarrow \left[ \begin{array}{cc\vert c} a_{11} & a_{12} &... ...{22}-a_{12}a_{21}} & \displaystyle{a_{11}b_{2}-b_{1}a_{21}} \end{array} \right]$$ (535)

$$-a_{12}/(a_{11}a_{22}-a_{12}a_{21})$$ (536)

$$\longrightarrow \left[ \begin{array}{cc\vert c} a_{11} & 0 & \dis... ...{22}-a_{12}a_{21}} & \displaystyle{a_{11}b_{2}-b_{1}a_{21}} \end{array} \right]$$ (537)

$$-1/a_{11}$$ (538)

$$-1/(a_{11}a_{22}-a_{12}a_{21})$$ (539)

$$\longrightarrow \left[ \begin{array}{cc\vert c} 1 & 0 & \displays... ...rac{a_{11}b_{2}-b_{1}a_{21}}{a_{11}a_{22}-a_{12}a_{21}}} \end{array} \right]\,.$$ (540)

ここで $a_{11}\neq0$ と

$$a_{11}a_{22}-a_{12}a_{21}\neq0$$ (541)

を条件としてかした． このとき拡大係数行列の階数は $2$ であり， 一意な解

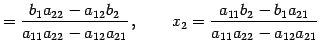$$x_{1} = \frac{b_{1}a_{22}-a_{12}b_{2}}{a_{11}a_{22}-a_{12}a_{21}}\,, \qquad x_{2}= \frac{a_{11}b_{2}-b_{1}a_{21}}{a_{11}a_{22}-a_{12}a_{21}}$$ (542)

をもつ． この結果より，行列 $A$ に対してスカラー量 $\det(A)$ を

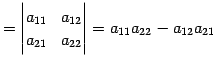$$\det(A) = \begin{vmatrix}a_{11} & a_{12} \\ a_{21} & a_{22} \end{vmatrix}= a_{11}a_{22}-a_{12}a_{21}$$ (543)

と定義する． $\det(A)$ を行列式（determinant）という． 以上より， 連立方程式の解の判別条件を得る． $\det(A)\neq0$ のとき行列 $A$ はフルランクであり 一意な解をもつ． $\det(A)=0$ のとき行列 $A$ はランクが落ち 一意な解をもたない．

同様にして正方行列 $A$ に対して行列式を定義すると

$$1\times1 \quad \det(A) = \begin{vmatrix}a_{11} \end{vmatrix}= a_{11}$$ (544)

$$2\times2 \quad \det(A) = \begin{vmatrix}a_{11} & a_{12} \\ a_{21} & a_{22} \end{vmatrix}= a_{11}a_{22}-a_{12}a_{21}$$ (545)

$$3\times3 \quad \det(A) = \begin{vmatrix}a_{11} & a_{12} & a_{13} \\ a_{21} & a_{22} & a_{23} \\ a_{31} & a_{32} & a_{33} \end{vmatrix}$$ (546)

$$= a_{11}a_{22}a_{33} -a_{11}a_{23}a_{32} +a_{12}a_{23}a_{31}$$ (547)

$$\quad -a_{12}a_{21}a_{33} +a_{13}a_{21}a_{32} -a_{13}a_{22}a_{31}$$ (548)

となる． 一般に $n\times n$ 行列では

$$\det(A)= \sum_{(k_{1},\cdots,k_{n})\in S_{n}}(\pm) a_{1,k_{1}}a_{2,k_{2}}a_{3,k_{3}}\cdots a_{n,k_{n}}$$ (549)

となることが予想される． ここで $k_{1},k_{2},\cdots,k_{n}$ は $1$ から $n$ の整数でお互いが異なる値をとる． 総和 $\sum$ はこの組合わせの全ての和をとる． 互いに異なる $n$ 個の組合わせを考えるので 足し合せる項は $n!$ である． すなわちこの組合わせの集合 $S_{n}$ は

$$S_{1} = \left\{ (1) \right\}\,,$$ (550)

$$S_{2} = \left\{ (1,2), (2,1) \right\}\,,$$ (551)

$$S_{3} = \left\{ (1,2,3), (1,3,2), (2,3,1), (2,1,3), (3,1,2), (3,2,1) \right\}\,$$ (552)

である． $S_{n}$ の元の個数は順列組合わせの個数となるので $n!$ 個である． 符合 $\pm$ は次節の置換の符合から定まる．

Kondo Koichi
Created at 2004/11/26