Next: 12 定積分と不定積分 Up: 6 積分法 Previous: 10 定積分 Contents
11 定積分の性質
定理 6.37 (定積分の性質)
定積分は次の性質をもつ:
- (1)
-
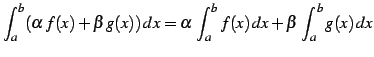 .
.
- (2)
-
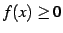
 のとき
のとき
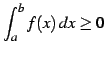 .
.
- (3)
-
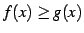
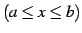 のとき
のとき
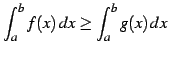 .
.
- (4)
-
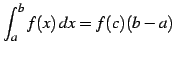 ,
,
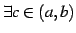 .
.
- (5)
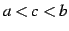 のとき
のとき
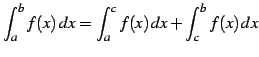 .
.
- (6)
-
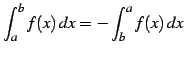 .
.
- (7)
-
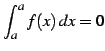 .
.
Kondo Koichi

Created at 2004/08/14